This paper is available on arxiv under CC 4.0 license.
Authors:
(1) A. Oliveros, Programa de F´ısica, Universidad del Atl´antico;
(2) Mario A. Acero, Programa de F´ısica, Universidad del Atl´antico.
Table of Links
- Abstract and Intro
- The f(Q) gravity: a brief review
- Cosmological dynamics in late-time
- Parameter constraints
- Conclusions
- Acknowledgments and References
3. Cosmological dynamics in late-time
In this section, we implement the above results taking into account a particular choice for f(Q), and study the resulting late-time cosmological evolution at the background level. To begin with, we introduce the f(Q) gravity model, which plays a central role in this work:
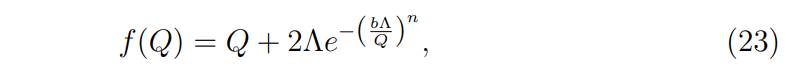
where Λ is the cosmological constant, and b and n are real dimensionless parameters. This model is inspired in that studied in Refs. [47, 48, 49], but in the context of f(R) gravity. It is evident that for b = 0 the model given by Eq. (23) is equivalent to GR plus the cosmological constant. In particular, from the structure of this model, it can be seen as a perturbative deviation from the ΛCDM Lagrangian. In this sense, this model can be arbitrarily close to ΛCDM, depending on the parameter b. It should be highlighted that in the literature also other exponential f(Q) gravity models have been intensively studied (see e.g., Refs. [18, 24, 25, 26, 31, 32, 33, 36]).
Following the procedure carried out in Ref. [50], we rewrite Eq. (13) in terms of N = ln a
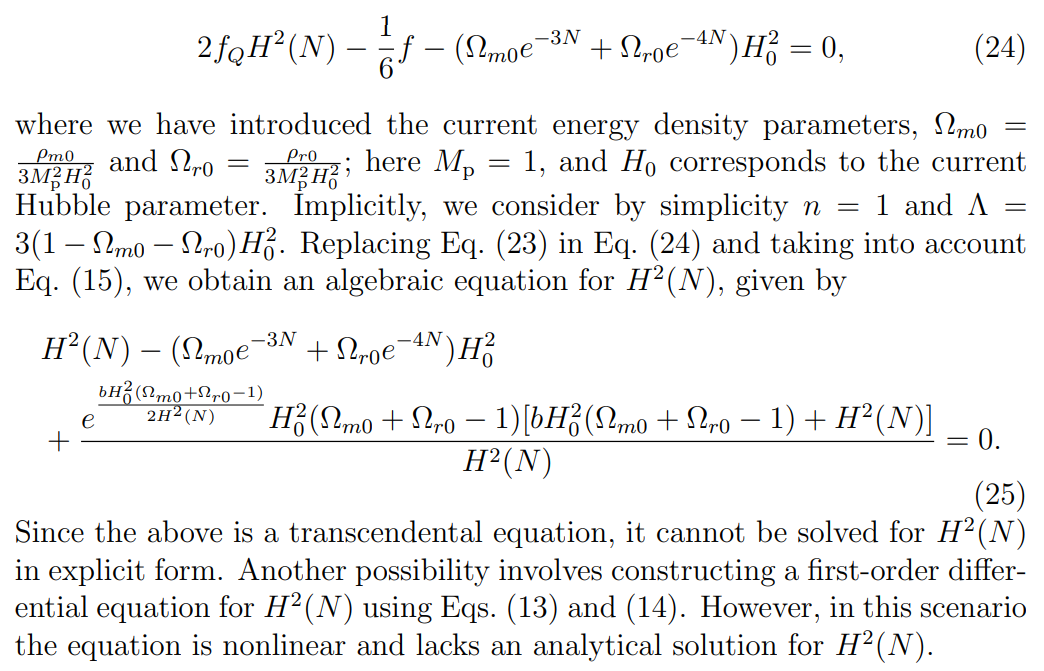
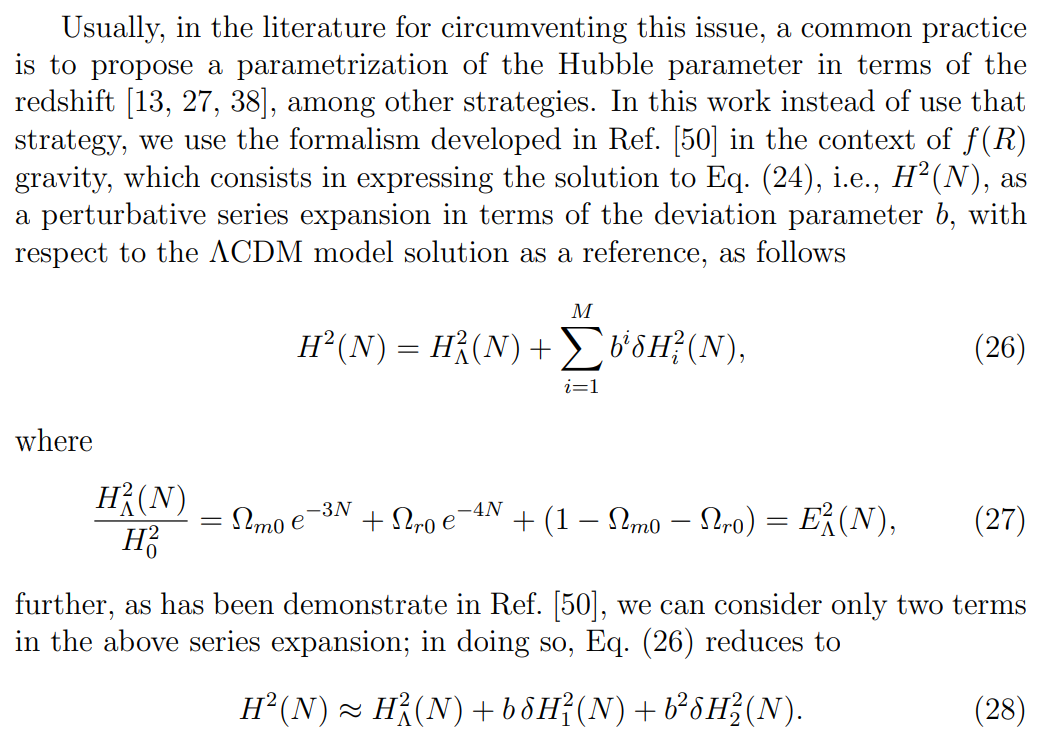
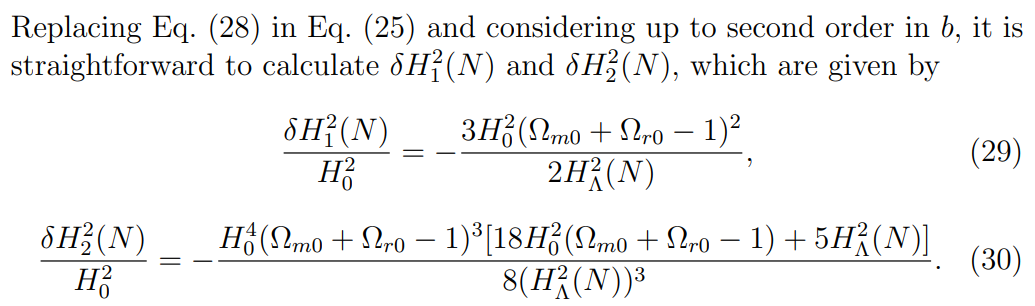
Now, replacing Eqs. (29) and (30) in Eq. (28), and using Eq. (27), we obtain an approximate solution for the Hubble parameter H(z):
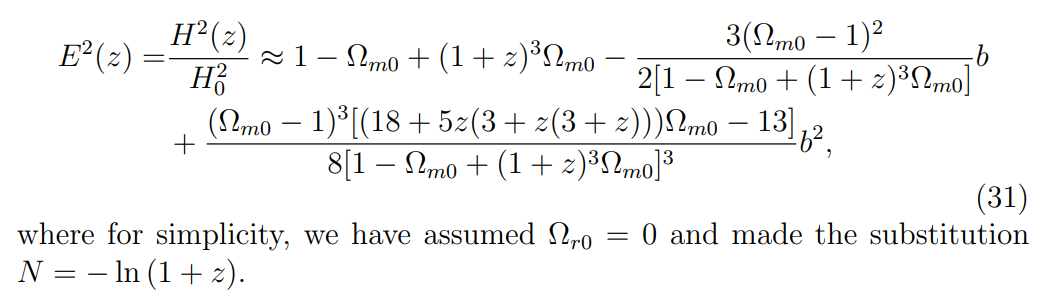
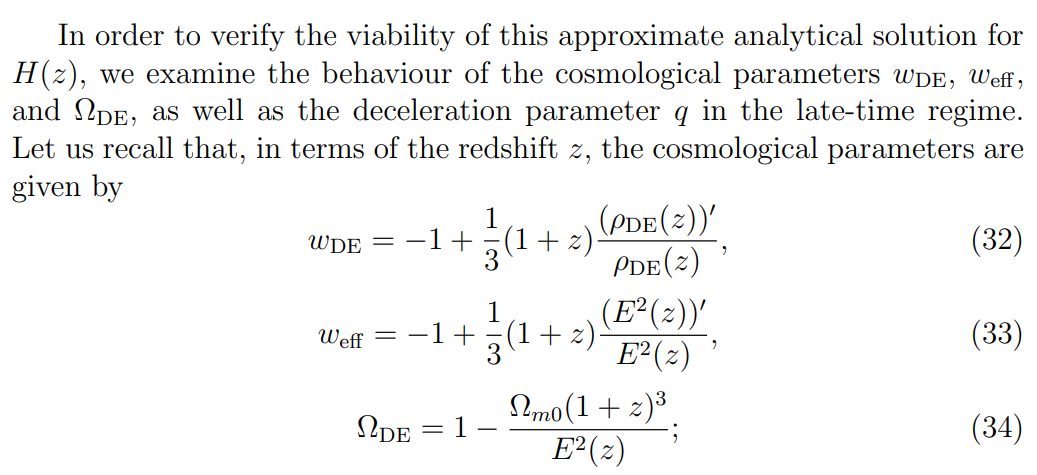
similarly, the deceleration parameter q is given by
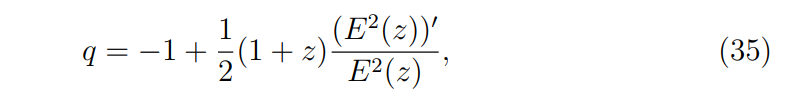
where the prime denotes differentiation with respect to z. Using Eqs. (19) and (31) and considering up to second order expansion in b, we obtain approximated analytical expressions for the above parameters in terms of the redshift z, as follows:
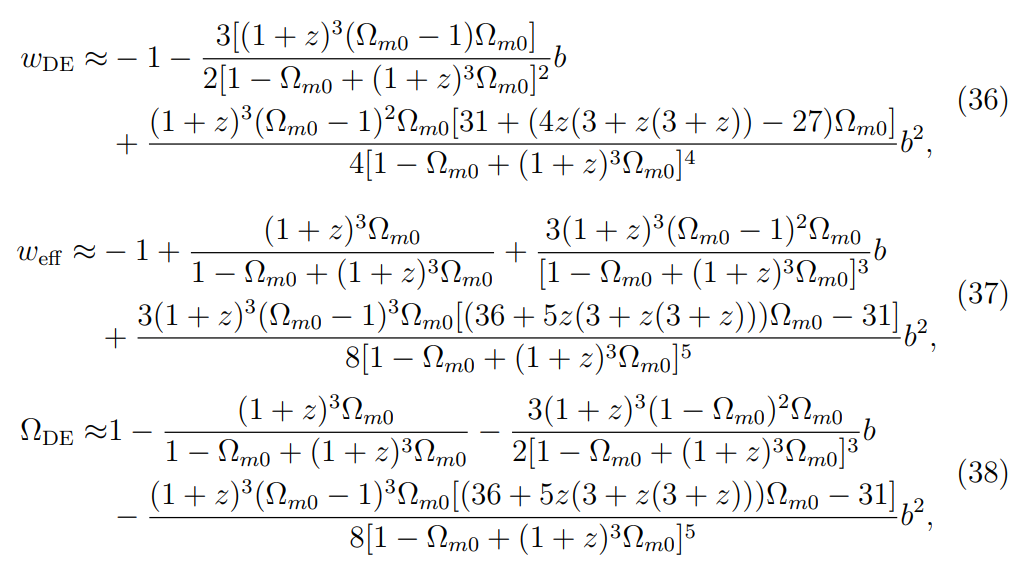
and
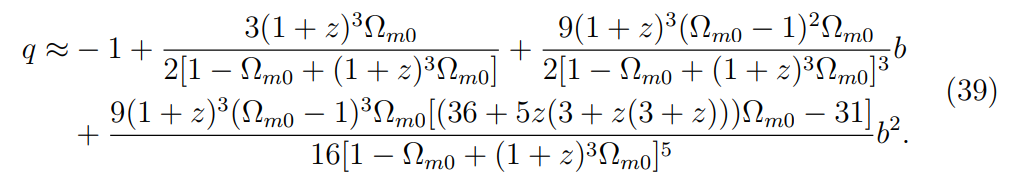
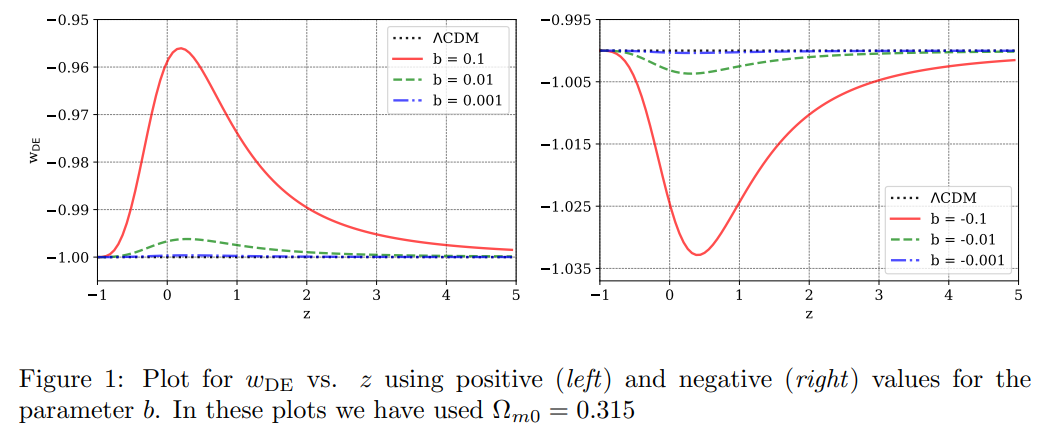
Notice that, as it would have been expected, the terms independent of b in each one of the last expressions correspond to those associate to ΛCDM model.
With Eqs. (36)-(39), we can plot the evolution of each parameter in terms of the redshift z. Additionally, in order to compare the results with the ΛCDM model, we have also incorporated in the corresponding plots the behavior associated with each quantity defined by Eqs. (32)-(35), but using Eq. (27) instead of (31).


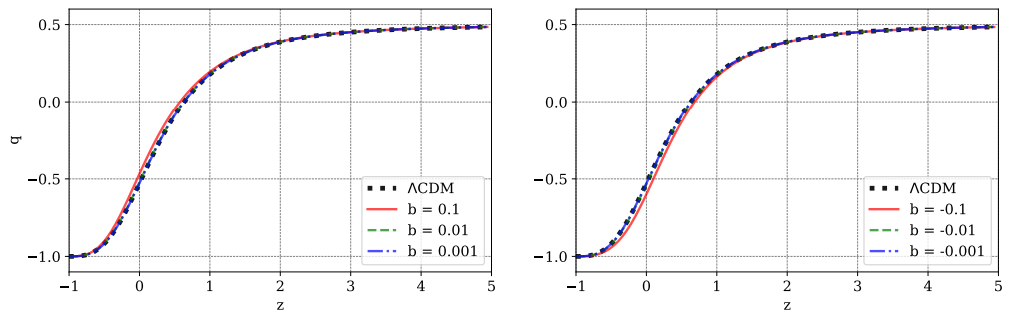
In general, from above we can deduce that as the magnitude of b increases, the present model deviates from ΛCDM model. This behavior is the expected, since by construction our approximated solution for H(z) is built as a perturbation of the ΛCDM model solution

