Author:
(1) CALLA TSCHANZ.
Table of Links
- Abstract and Intro
- Background on tropical perspective
- The expanded construction
- GIT stability
- Stack perspective
- The canonical moduli stack
- References
3. The expanded construction
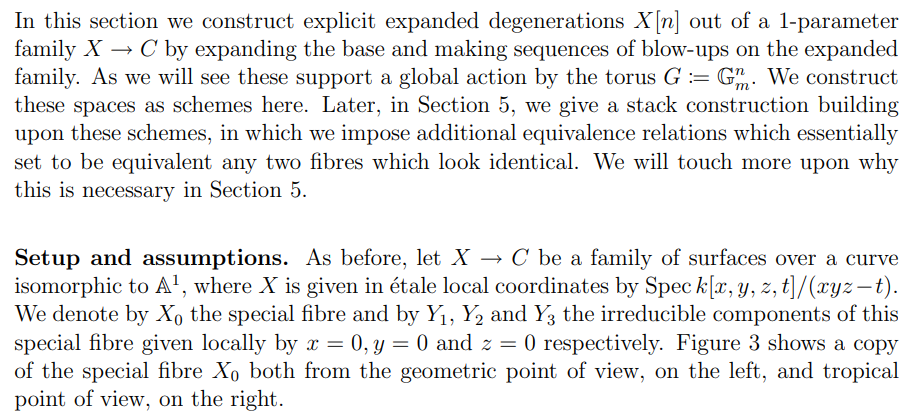
Output of expanded construction. The expanded degeneration X[n] ! C[n] which we construct in this section has the following properties:
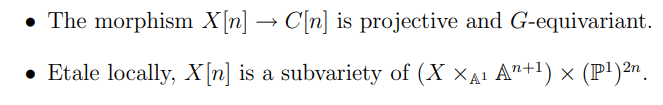
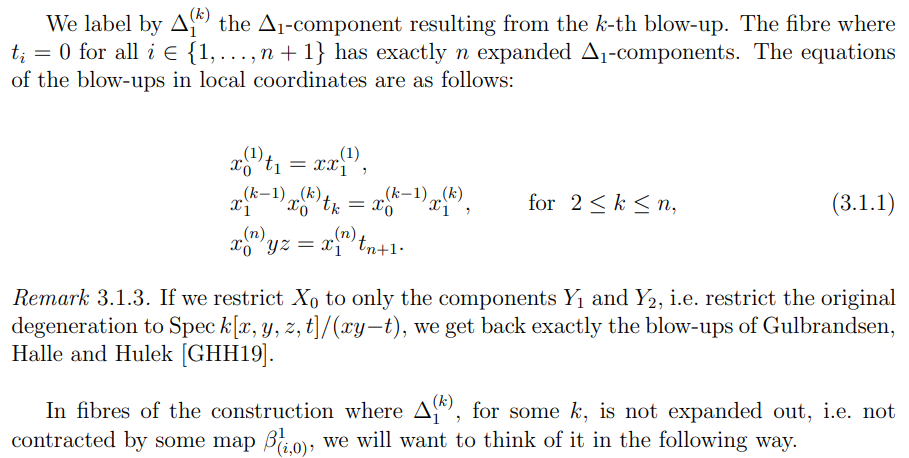
3.1 The blow-ups
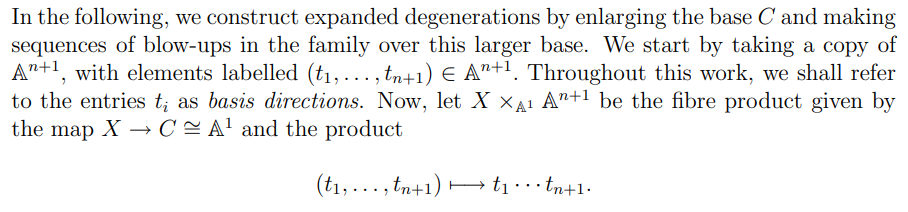
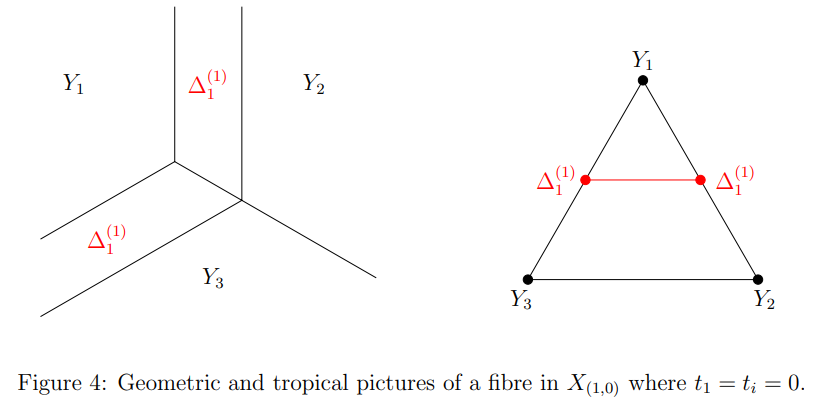
In this expanded degeneration construction, we will be blowing up schemes along Weil divisors. A consequence of the way these blow-ups are defined is that the blow-up morphisms contract only components of codimension at least 2.
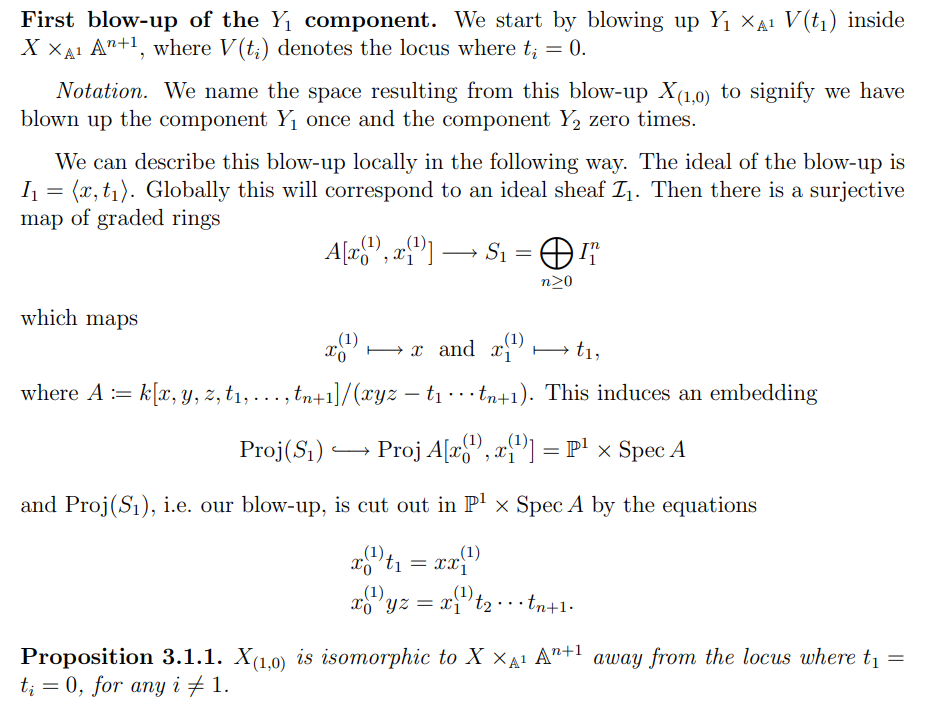
the morphisms corresponding to each individual blow-up. We therefore have the equality
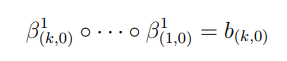
We now fix the following terminology.

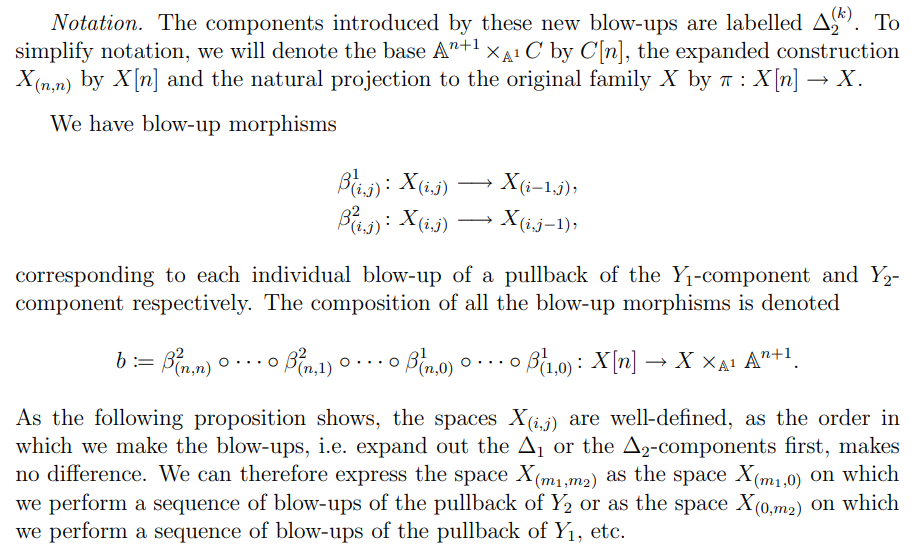
Proposition 3.1.5. The following blow-up diagram commutes
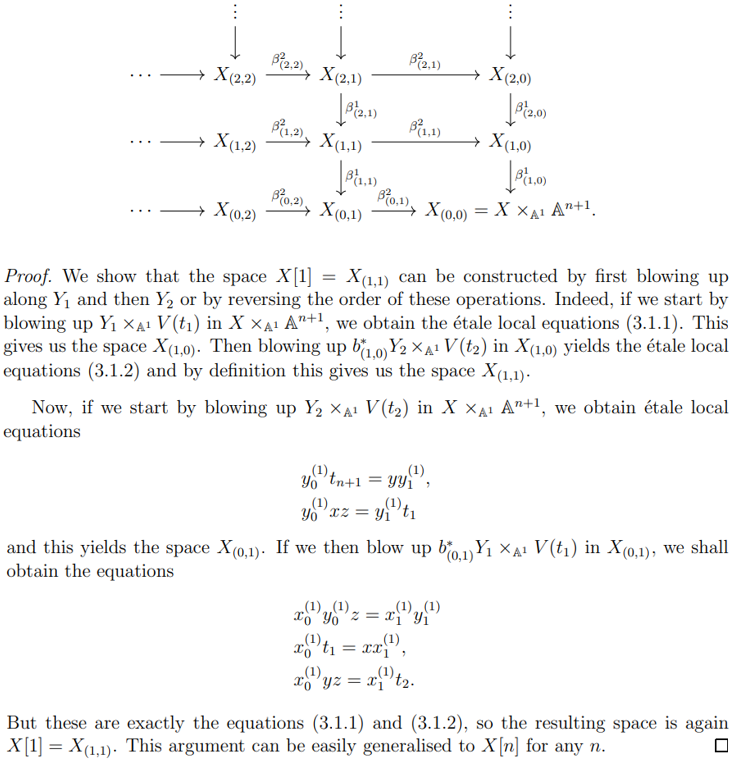

Proof. This is immediate from the local description of the blow-ups above.
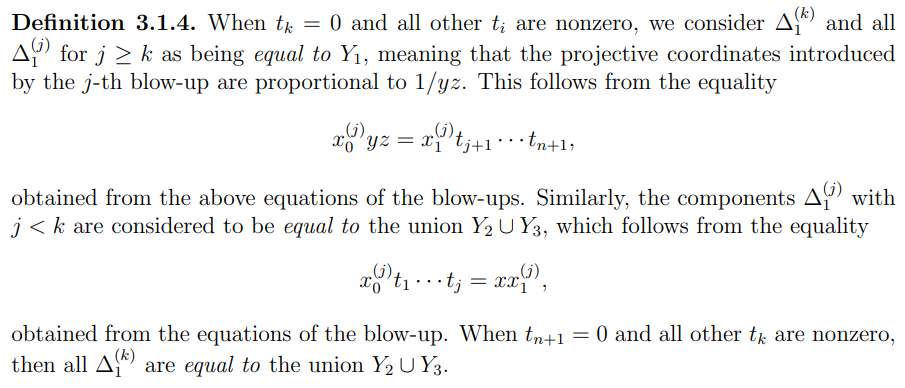
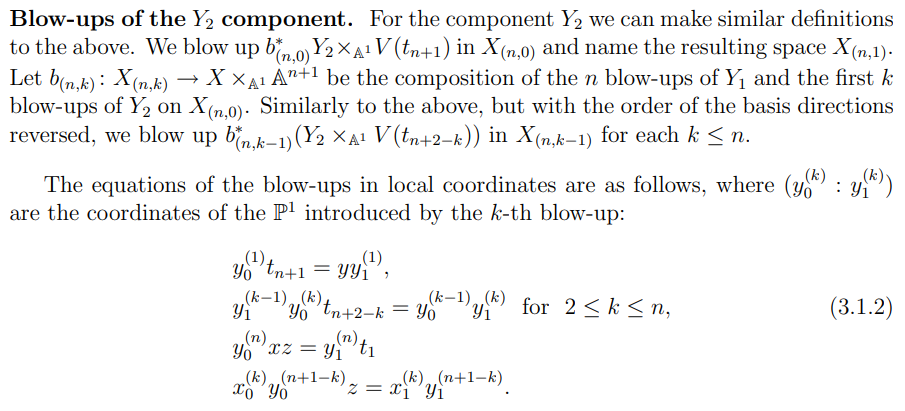
We now extend the definition of ∆1-components to the schemes X[n] and fix some additional terminology.
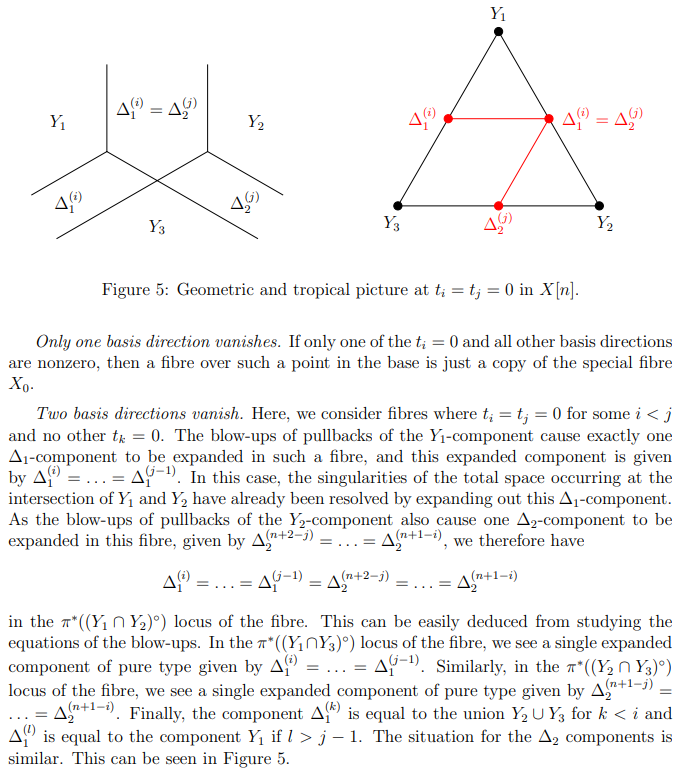
Before we continue we fix some terminology which will help us describe the expanded components.
Definition 3.1.11. We refer to an irreducible component of a ∆-component as a bubble. The notions of two bubbles being equal and a bubble being expanded out in a certain fibre are as in Definitions 3.1.4 and 3.1.9.
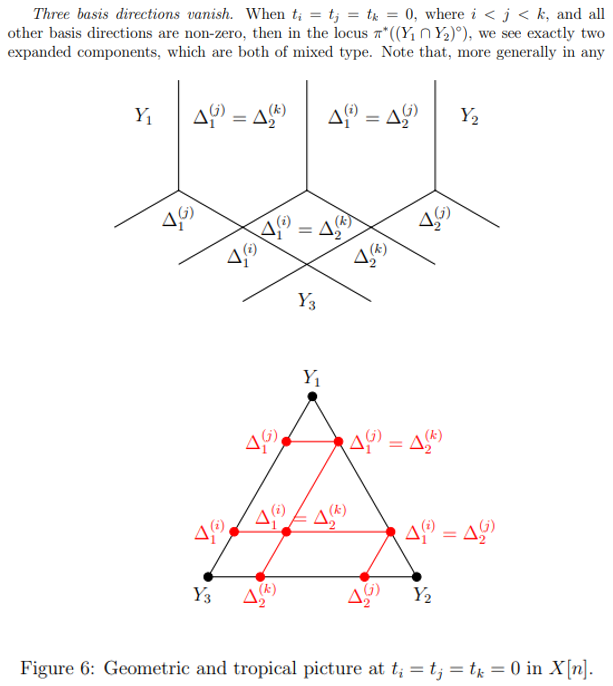
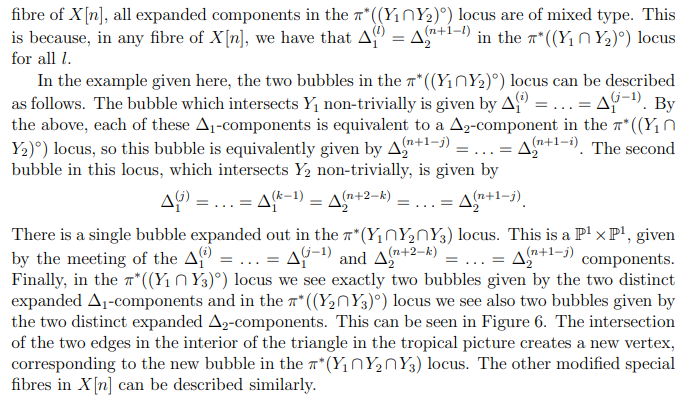
Now, we note that there is a natural inclusion
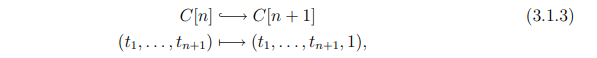
which, in turn, induces a natural inclusion
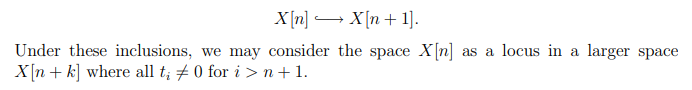

on the basis directions, and acts by
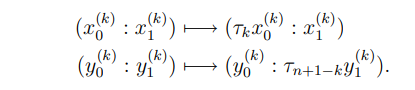
on the ∆-components.
Proof. This follows immediately from [GHH19].
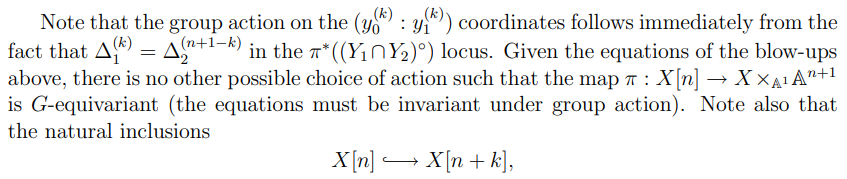
we described in the previous section are equivariant under the group action.
Lemma 3.1.13. We have the isomorphism
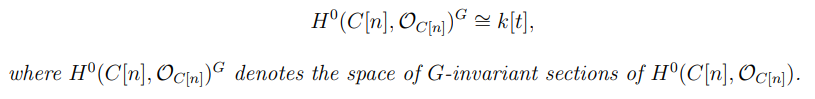
Proof. This is immediate from the above description of the group action.
Remark 3.1.14. We abuse notation slightly by referring to the group acting on X[n] by G, instead of G[n]. It should always be clear from the context what group G is meant.
3.2 Embedding into product of projective bundles
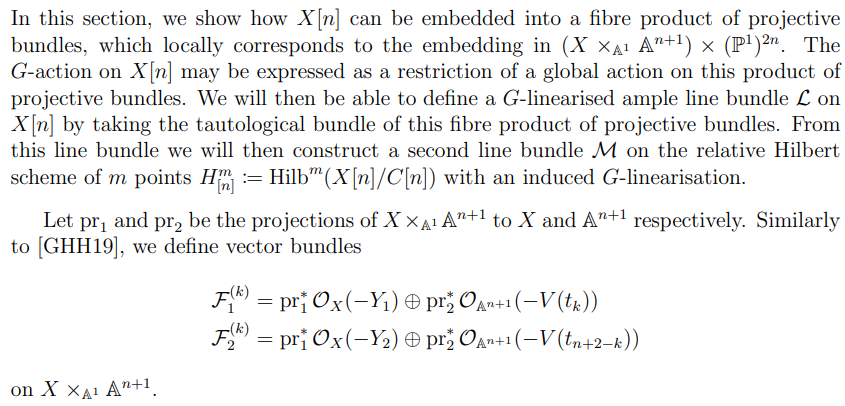
Lemma 3.2.1. There is an embedding
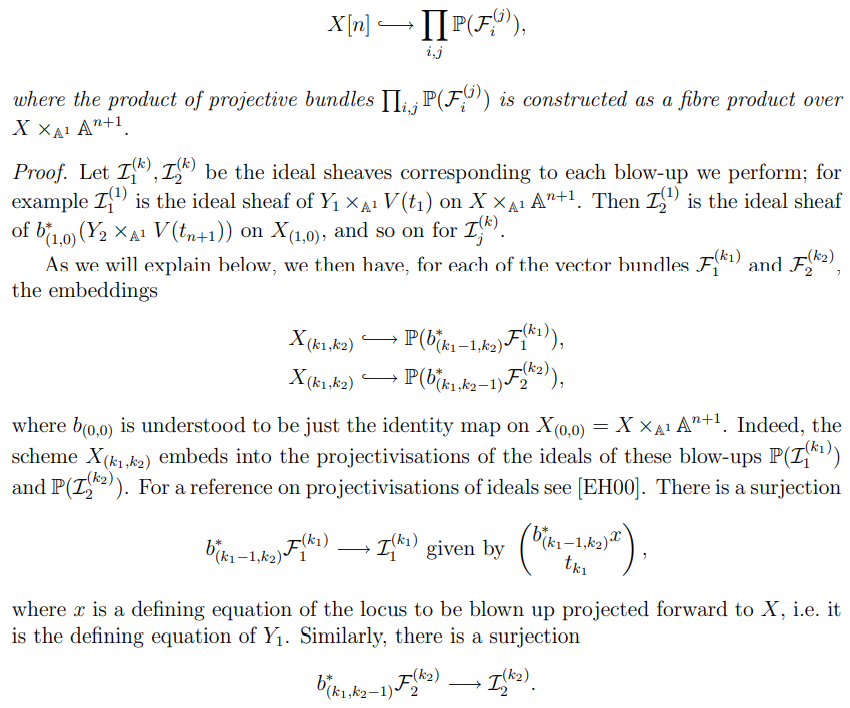
From this, we deduce that there are embeddings
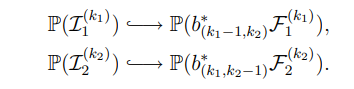
Hence we have embeddings
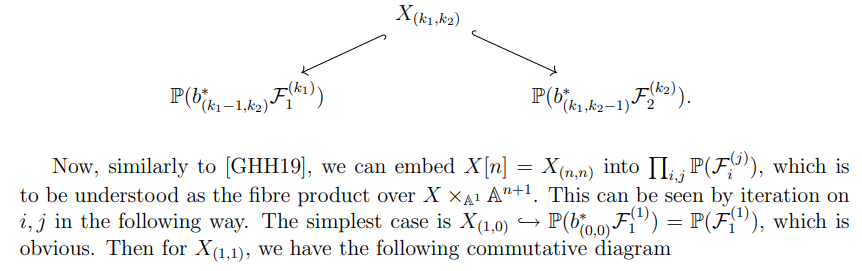
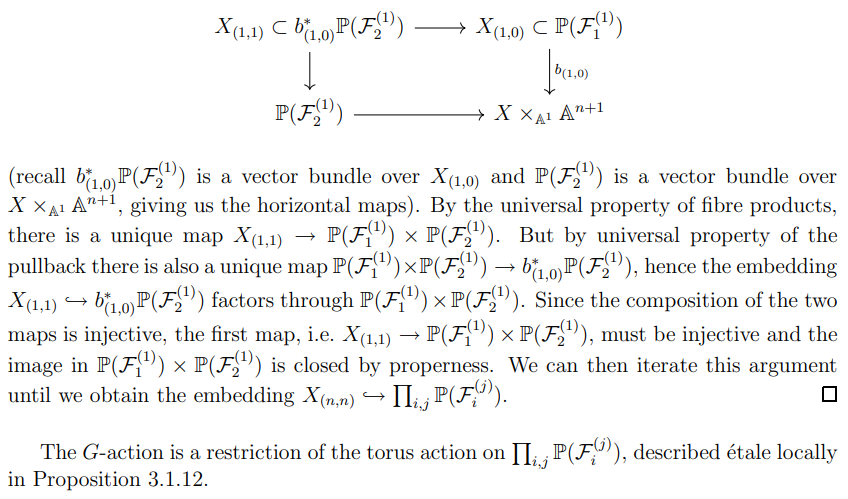
Linearisations. The following lemma gives a method to construct all the linearised line bundles we will need to vary the GIT stability condition.

This paper is available on arxiv under CC 4.0 license.

